$>$ How to divide fractions and mixed numbers word problems

$Lesson summary$

Get more contents on this skill...

Hey kids, learn how to divide fractions and mixed numbers word problems using this step-by-step technical guide. This guide will equally offer fifth graders strategic ways to reason and tackle word problems on dividing fractions and mixed numbers.

We promise you that your kid’s fractions skills will enhance as they strive to have constant practice with this step-by-step guide. Hence, solving complex mixed numbers word problems will be a piece of cake for them.

Besides, this guide will encourage kids to follow a general strategy instruction method of dividing fractions and mixed numbers word problems wherein they’ll have to;

Read the problem in question to understand it very well,
Devise a good plan or have a good mental representation of the question,
Provide a solvable math equation related to the problem in question,
Check their work thoroughly once more to ensure that their solution is accurate.

With this word problem-solving approach, we bet your kids can divide fractions and mixed numbers word problems perfectly.

Steps on how to solve division of fractions and mixed numbers word problems

Found below here are simple steps on how to solve division of fractions and mixed numbers word problems. Since fractions and mixed numbers word problems are always tricky, we will devise simple steps to do it effortlessly.

These steps are well-organized to help grade 5 kids tackle and conceptually understand the operations very well. Most importantly, kids will learn how to use logical reasoning to predict or estimate answers, which is vital for their brain development.

Also, to enhance a perfect understanding of the above steps, we have added real-life examples to show kids how these steps work.

Step 1: IDENTIFY THE PROBLEM:

To identify the problem, you must read the word problem very carefully to know what the problem wants you to solve. Does the problem need you to divide or perform any other operation?

You can answer this question by identifying the keywords in the word problem. For instance, if it is a division operation, then you’ll see the following keywords:- divide, half, evenly, every, per, parts, out of, quotient of, ratio of, how many in each, equal parts, cut up, average, as much, etc.

***One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***

Step 2: STRATEGIZE OR GATHER RELEVANT INFORMATION:

How do you tackle this problem?

First, from the keyword(s) in the word problem, you will know whether you need to perform a Division Operation or any other operation.
On the other hand, you must not count only on keywords. Always try to understand the situation that the problem is describing.
After knowing which operation you will perform, build short sentences to represent the given word problem.

Step 3: SET UP AN EQUATION:

Now, write down a numerical equation representing the information given in the word problem.

Step 4: PROVIDE A SOLUTION:

From step 3 above;

Firstly, if the word problem involves a whole number, you will need to convert the whole number into a fraction by dividing the whole number by 1.
Secondly, if the word problem involves a mixed number, you will need to convert the mixed number into an improper fraction.
Thirdly, in dividing fractions, the easiest way to solve is to invert the second fraction and then rewrite the division problem as a multiplication problem.
Fourthly, multiply the numerators across and also multiply the denominators across. Note that when multiplying fractions, a common denominator is not needed.
Fifthly, simplify the fraction if possible.
Lastly, do not forget to add the unit to measurement to the final result, if any.

Step 5: CHECK YOUR WORK:

Finally, check if your answer makes sense. For instance, estimate the answer and see if it is close to what you expected. However, if the answer is not what you expected, go back to step one and start all over again.

$Find more resources here$

PRACTICE THIS SKILL NOW $Play$

Download worksheet

Dividing fractions and mixed numbers

$Dividing fractions and mixed numbers worksheet$

Print it

Examples on how to divide fractions and mixed numbers word problems.

Example one

Yesterday, Peter brought 50 boxes of vegetable oil to distribute at a family reunion. If he gave each person a box of vegetable oil, how many people were at the family reunion?

Step 1: First, the important fraction and whole numbers here are 50 and . The keyword found in the word problem is “each.”

Step 2: Next, how will you solve the problem? After reading and understanding the word problem very well, you’ll see that the situation the problem is describing, and the keyword(s) found call for you to perform a division operation.

Now, set up short sentences to represent the given word problem.

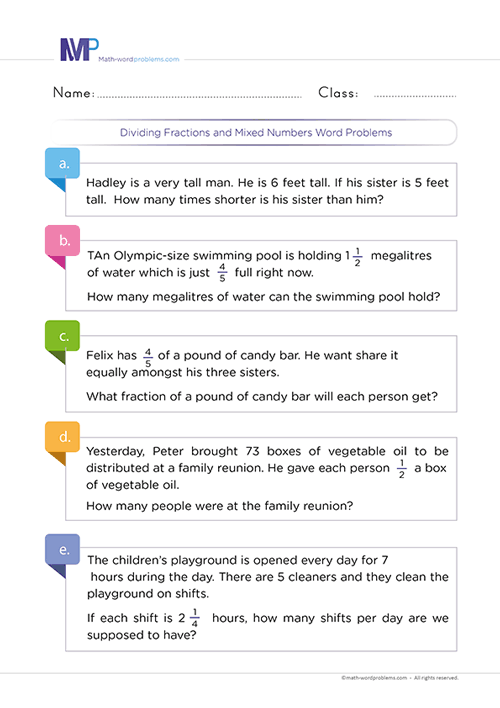
Number of boxes of vegetable oil Peter brought = 50 boxes
Number of boxes of vegetable oil he gave each person = a box
Therefore, the number of people at the reunion = the number of boxes of vegetable oil Peter brought ÷ the number of boxes of vegetable oil he gave each person

Step 3:Now, write down a numerical expression to represent the bolded sentence in step 2 above to solve this word problem:

50 ÷ = ?

Step 4:After that, from Step 3 above:

Since the word problem involves a whole number and a fraction, you will need to convert the whole number into a fraction by dividing the whole number by 1.
Then, invert the second fraction and rewrite the division problem as a multiplication problem.
Now, multiply the numerators across and also multiply the denominators across. Note that when multiplying fractions, a common denominator is not needed.
Also, simplify the fraction if possible.
Furthermore, always add the unit of measurement to the final result, if any.

So, there were 100 people at the reunion.

Step 5: Finally, check if your answer makes sense. For example, estimate the answer and see if it is close to what you expected. However, if the answer is not what you expected, go back to step one and start all over again.

Example two

The children’s playground is open every day for hours during the day. There are 5 cleaners, and they clean the playground on shifts. If each shift is hours, how many shifts are there per day?

Step 1: First, after reading and discarding irrelevant information in the word problem, you see that the important mixed numbers here are and and the most important keyword found in the word problem is “per.”

Step 2:How will you solve the problem? The situation, the problem is describing, and the keyword calls for you to perform a division operation.

Now, construct short sentences to represent the given word problem.

Number of hours the children’s playground is open every day =
Number of hours each shift last =
Therefore, the number of shifts per day = the hours the children’s playground is open every day × the hours each shift lasts.

Step 3:Then, write down a numerical equation for each bolded sentence in step 2 above to solve this word problem:

÷ = ?

Step 4: From step 3 above:

Since the word problem involves mixed numbers, you need to convert the mixed numbers into an improper fraction.
Secondly, in dividing fractions, the easiest way to solve is to invert the second fraction and then rewrite the division problem as a multiplication problem.
Thirdly, you are going to multiply the numerators across and also multiply the denominators across. Note that when multiplying fractions, a common denominator is not needed.
Fourthly, simplify the fraction if possible.
Finally, add the unit of measurement to your final answer, if any.

So, there are shifts per day.

Step 5: Lastly, check if your answer makes sense. For example, estimate the answer and see if it is close to what you expected. However, if the answer is not what you expected, go back to step one and start all over again.

Example three

If liters of olive oil are distributed equally into 11 containers. How many liters are in each container?

Step 1: First, the important mixed number and whole number here are and 11. The most important keyword found in the word problem is “equally.”

Step 2:Next, how will you solve the problem? You see that the situation the problem is describing and the keyword found in the word problem calls for you to perform a division operation.

Now, construct short sentences to represent the given word problem.

Total liters of olive oil = liters
Number of containers that the olive oil is distributed into = 11
Therefore, the liters of olive oil per container = the total liters of olive oil ÷ the number of containers the olive oil is distributed into.

Step 3:Then, write down a numerical equation for each bolded sentence in step 2 above to solve this word problem:

÷ 11 = ?

Step 4: From step 3 above:

First, since the word problem involves a whole number, you will need to convert the whole number into a fraction by dividing the whole number by 1..
Next, since the word problem involves a mixed number, you will need to convert the mixed number into an improper fraction.
Now in dividing fractions, the easiest way to solve is to invert the second fraction and then rewrite the division problem as a multiplication problem.
After that, multiply the numerators across and also multiply the denominators across. In addition to this, when multiplying fractions, a common denominator is not needed.
Furthermore, simplify the fraction if possible.
Last but not the least, do not forget to add the unit of measurement to the final result, if any.

So, there are liters of olive oil in each container.

$>$ How to divide fractions and mixed numbers word problems

Get more contents on this skill...