Best strategy to use to understand fractions word problems
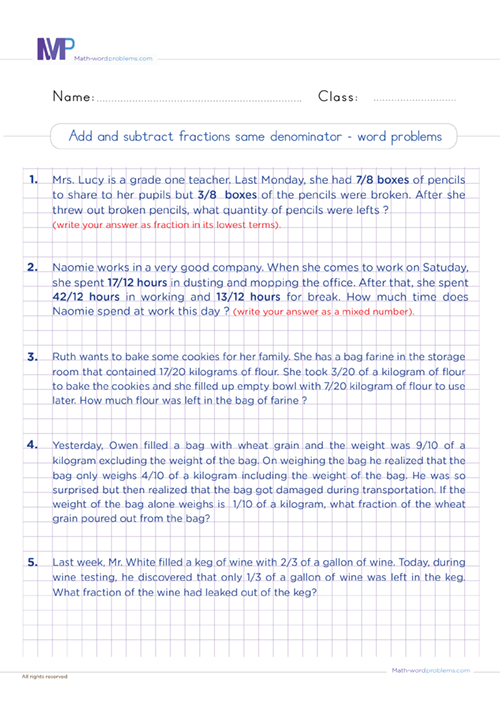
Best strategy to use to understand fractions word problems

- INTRODUCTION
- Step1 Identify
- Step 2 STRATEGIZE
- Step 3 SET UP
- Step 4 PROVIDE A SOLUTION
- Step 5 CHECK YOUR WORK
- Examples Example on understanding fractions
Get more contents on this skill...
Grab here the best strategy to use to understand fractions word problems. These strategies will stimulate kids’ minds with a better understanding of how to read a word problem, then reason out that it is an understanding fractions word problem.
Also, we consider this strategy the best because it will ignite kids’ mental representation and reading comprehension skills, enhancing a successful fractions word problem-solving process.
Furthermore, this strategy will provide sixth graders with explicit instruction in the conceptual knowledge and problem schemes that we need to solve complex math word problems to understand fractions.
Simple steps to solve understanding fractions word problems
As earlier said, your kids will be excited using simple steps to solve understanding fractions word problems. These steps will enable your kids to apply their representational and reading comprehension skills, which are essential when solving fraction word problems.
In addition, we will include all the conceptual key features you’ll need to tackle all fractions word problems. This ensures that kids pay more attention when they face challenging word problems and have excellent organizational skills. Thus, greatly uplifting their working memory.
Most importantly, a challenging yet fun real-life example will be attached below to understand better how this strategy works for kids to follow through.
Step 1: IDENTIFY
To begin, you have to read the word problem very well while trying to figure out the important numbers and keywords in the word problem.
After that, you can now use these keywords to tell if the problem has to be solved as a fraction.
- That said, if the problem is understanding fractions, the only common keyword you need to look out for is “What fraction of.” So the word problem must consist of a part and a whole.
Note: One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use
Step 2: STRATEGIZE
How will you solve or tackle the word problem?
As mentioned above, to be able to understand fractions, you have to understand some vocabulary used in fractions, such as:
- The part, also known as the numerator, is the top number in a fraction. The numerator tells us how many pieces we are dealing with.
- The whole, also known as the denominator, is the bottom of a fraction. The denominator describes the size of the pieces.
- Again, know that you must not rely merely on keywords. Rather make sure you understand the situation that the problem is describing very well before you dive into solving it.
- Therefore, after knowing what the part (numerator) and the whole (denominator) will be, construct short expressions/sentences to represent the given word problem.
Step 3: SET UP
Now, write down a numerical expression representing the information given in the word problem. Use “/” to separate the numerator from the denominator.
Step 4: PROVIDE A SOLUTION
From step 3 above, simplify the fraction if need be. Most importantly, always include the unit of measurement in your final answer, if any.
Step 5: CHECK YOUR WORK
Lastly – Ask yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.
Example on understanding fractions
Example one solution a
- What fraction of the cake did James eat?
- What fraction of the cake was left?
Step 1: Frist, from careful reading and analysis of the problem, the important numbers here are 12, which is the whole, and 3, which is the part. So, the keyword in the word problem is “what fraction of. “
Step 2: How will you solve or tackle the word problem?
It is clear that from the situation the problem is describing, the keyword found in the word problem and what we gathered from step 1 above shows that this is an understanding of fractions problem.
Therefore, since you already know the part (numerator) and the whole (denominator), construct short expressions/sentences to represent the given word problem.
- Pieces of cake that James ate = 3
- Total number of pieces that the cake was divided into = 12
- Therefore, the fraction of cake James ate = pieces of the cake that James ate as the numerator, and the total number of pieces that the cake was divided into as the denominator.
Step 3: Write down a numerical fraction to represent the bolded statement in step 2 above. Use “/” to separate the numerator from the denominator.
Step 4:
So James ate
Step 5: Finally, check your work by asking yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.
Solution b
Step 1: Frist, from carefully reading and analyzing the problem, you will understand that this question involves so many steps. You have to read it between the lines to grasp what you need to do.
For instance:
- You need to know the total pieces of the cake they ate, which calls for an addition operation.
- Then you need to know the pieces of cake left, which calls for a subtraction operation.
- However, the part is not clearly given here, so you have to figure it out during your solution process, but the whole is 12.
- Also, the keyword in the word problem is “what fraction of.”
Step 2: How will you solve or tackle the word problem?
It is clear that from the situation the problem is describing, the keyword found in the word problem and what we gathered from step 1 above shows that this is an understanding of fractions problem.
Therefore, since you already know what the whole (denominator) is from step 1 above and is going to figure out what the part (numerator) later, construct short expressions/sentences to represent the given word problem
- Pieces of cake that James ate = 3
- Pieces of the cake that the birthday girl ate = 5
- Total pieces of the cake that they both ate = 3 + 5 = 8
- Total number of pieces that the cake was divided into = 12
- Pieces of the cake left = Total number of pieces that the cake was divided into - Total pieces of the cake that they both ate
→ 12 – 8 = 4 → here is the part
- Therefore, the fraction of cake left = pieces of the cake left as the numerator and the total number of pieces that the cake was divided into as the denominator.
Step 3: Write down a numerical fraction to represent the bolded statement in step 2 above. Use “/” to separate the numerator from the denominator.
Step 4:From step 3 above, simplify the fraction if need be. In addition to this, always make sure to include the unit of measurement in your final answer, if any.
So,
Step 5: Finally, check your work by asking yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.