How to carry out understanding fractions as division word problems?
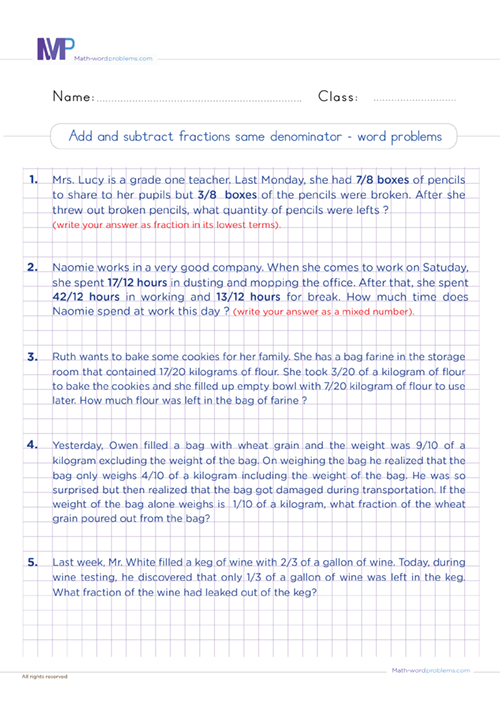
How to carry out understanding fractions as division word problems?

- INTRODUCTION
- Step1 Identify
- Step 2 STRATEGIZE
- Step 3 SET UP
- Step 4 PROVIDE A SOLUTION
- Step 5 CHECK YOUR WORK
Get more contents on this skill...
Here are captivating steps on how to carry out understanding fractions as division word problems. These steps will enhance your kid’s flexibility and reasoning skills when solving fraction problems.
One interesting thing to note about our understanding of fractions as division problems is how it relates the literature in the word problem with the mathematical concept in that particular word problem. As such, kids will no longer face difficulties when translating the literature part of the word problem into maths equations.
Easy steps to solve understanding fractions as division word problems
Below here are easy steps to solve understanding fractions as division word problems. You’ll enjoy solving using the technical tips embedded in each step. As a matter of fact, your kids will come across the best and quick strategy for solving complex word problems.
Also, the difficulties your kids usually face when creating complete and correct mental problem representations have been taken care of in the following steps. In other words, after learners go through these steps, they will be able to brush through any complex word problems.
To demonstrate how excellent these steps work, we will solve some outstanding real-life examples below.
Step 1: IDENTIFY:
Here, after reading the word problem very carefully and discretely, look out for important numbers and keywords in the word problem.
Then, use these keywords to identify whether the problem involves understanding fractions as division or any other operation.
- So, in Understanding fractions as division, we look for common keywords such as divide, half, evenly, every, per, parts, out of, quotient of, ratio of, how many in each, equal parts, cut up, average, as much, etc.
- ***One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
- For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***
Step 2: STRATEGIZE:
As you move to this step, you need to ask yourself this question: - “How will I solve or tackle the word problem? “
So, to be able to understand fractions as division, you have to understand some vocabulary used in fractions, such as:
- First, the part, also known as the numerator, is the top number in a fraction. The numerator tells us how many pieces we are dealing with.
- Then, the whole, also known as the denominator, is the bottom of a fraction. The denominator describes the size of the pieces.
- But know that you must not rely entirely on keywords. You should read the problem to understand the situation that the problem is describing very well before you dive into solving it.
- So, after knowing what the part and the whole will be and the operation you need to perform with the help of keyword(s), construct short expressions/sentences to represent the given word problem.
Step 3: SET UP:
As we move to this step, write down a numerical expression representing the information given in the word problem.
Step 4: PROVIDE A SOLUTION:
From Step 3 above, use a division sign, preferably “/,” to separate the part from the whole. Furthermore, simplify the fraction if need be. Then, add the unit of measurement to your final answer if any
Step 5: CHECK YOUR WORK:
Finally, ask yourself this question. Does my answer make sense? If “YES”, you are done. If “NO”, go back to step 1 and start all over again.
Example on understanding fractions as division word problems
Step 1: After reading the question discretely, you see that the important numbers here are 30, which is the whole, and 8, which is the part. Also, the keywords found in the word problem are “divide” and “equally.”
Step 2:Now, how will you solve the problem? As you can see, from the situation that the problem describes, the keyword found in the word problem and what we gathered from step 1 above indicate that this is understanding of fractions as a division problem.
Since you already know what the part and the whole is and the operation you need to perform, you can now go ahead and construct short expressions/sentences to represent the given word problem.
- Number of bowls she divided the icing sugar into = 8.
- Total quantity of icing sugar Hanna has = 30.
- Therefore, the amount of icing sugar in each bowl = the number of bowls she divided the icing sugar into ÷ the total quantity of icing sugar Hanna has.
Step 3:Then, write down a numerical expression to represent the bolded sentence in step 2 above:
8 30 = ?
Step 4: After that, from Step 3 above, use a division sign (this one “/” in particular) to separate the part from the whole. Furthermore, simplify the fraction if need be. Additionally, always remember to add the unit of measurement to your final answer, if any.
So, of the icing sugar was in each bowl.
Step 5:Finally, check your work – Ask yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.