Fractions and mixed numbers worksheet with answers grade 5
Fractions and mixed numbers worksheet with answers grade 5

- INTRODUCTION
- Download worksheets
- Related Contents
- Strategies for quickly mastering fractions and mixed numbers...
Get more contents on Fractions And Mixed Numbers Word Problems...
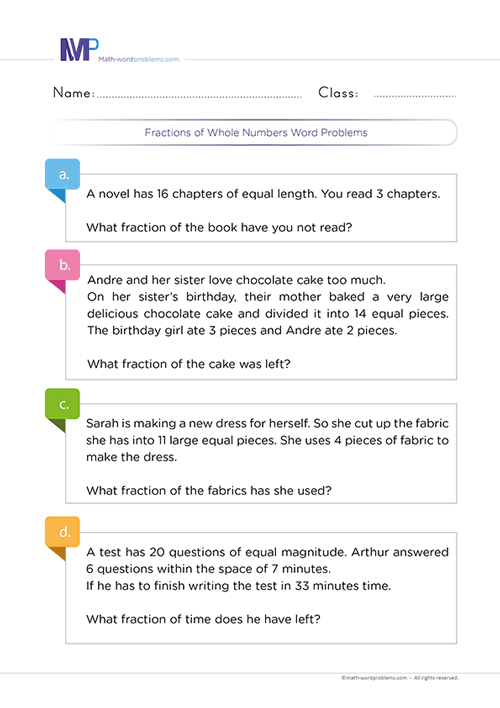
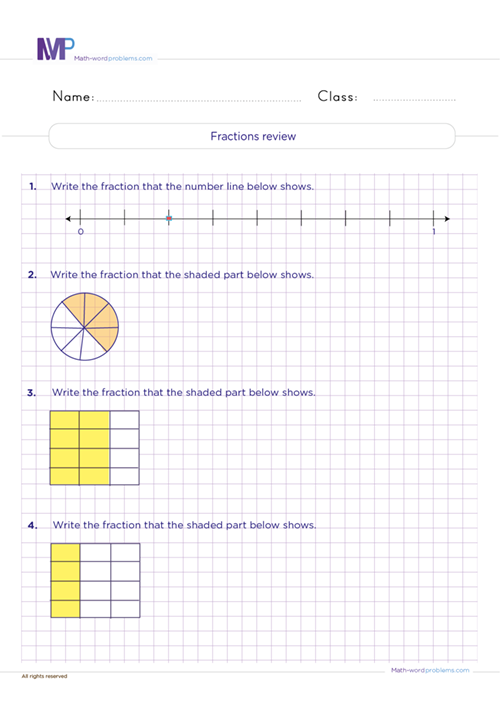
Fractions and mixed numbers worksheet with answers Grade 5 are designed to offer 5th graders simple ways of adding, subtracting, multiplying, and dividing mixed numbers. A fraction, in simple words, is part of a whole. For instance, if an orange is cut into 4 pieces to share with four kids, what quantity of orange will each child have? Each child will have ¼. This ¼ is called a fraction of a whole (I orange). Here, 1 is the numerator, while 4 is the denominator. So, for a number to be a fraction, it must consist of a numerator and a denominator.
On the other hand, a mixed number consists of a whole number and a fraction. For instance, 3 ½. From the example, you can see that a mixed number is a combination of a whole number (3) and a fractional part (½). Once you master these skills, you will enjoy solving our thrilling fractions and mixed numbers problems. Most people find it easy to work with mixed numbers, whereas others prefer to convert the mixed number to an improper fraction before working with them.
Thus, how do we convert a mixed number to an improper fraction? To convert a mixed number to an improper fraction (3 ½) ;
- first, multiply the whole number by the denominator 3 x 2 = 6
- add the product to the numerator 6 + 1 = 7
- finally, the sum (7) will become the new numerator, while the denominator (2) remains the same. So, 3 ½ converted to an improper fraction will be 7/2
unlike proper fractions whose numerators are always smaller than the denominators, the numerator of an improper fraction is always greater than the denominator.
As earlier said, we will provide plenty of fractions and mixed numbers worksheets wherein the exercises for each concept (adding, subtracting, multiplying, dividing, scaling fractions) will be formulated in its worksheet. This is so that kids should have accurate mastery of each exercise before moving on to the next. The exercises are short and easy for kids to solve in just one sitting.
Most importantly, our fractions and mixed numbers practice resource is given with a solution and answer sheet to help kids check their answers and do corrections in case of a mistake.
Adding, subtracting, multiplying, and dividing mixed numbers worksheet Grade 5
Our adding, subtracting, multiplying, and dividing mixed numbers worksheet Grade 5 offers an effective way with fun strategies of solving math operations on fractions efficiently. We will formulate a variety of simple exercises and real-life problem-solving on fractions and mixed operations for kids to practice how to add, subtract, multiply, and divide fractions in math class and real life. We have realized how challenging it usually is when we need to solve fraction problems in real life. To solve this problem, we designed an exceptional fractions and mixed numbers worksheet with answers Grade 5 for kids to learn and know how to add, subtract, multiply, and divide fractions problems effortlessly.
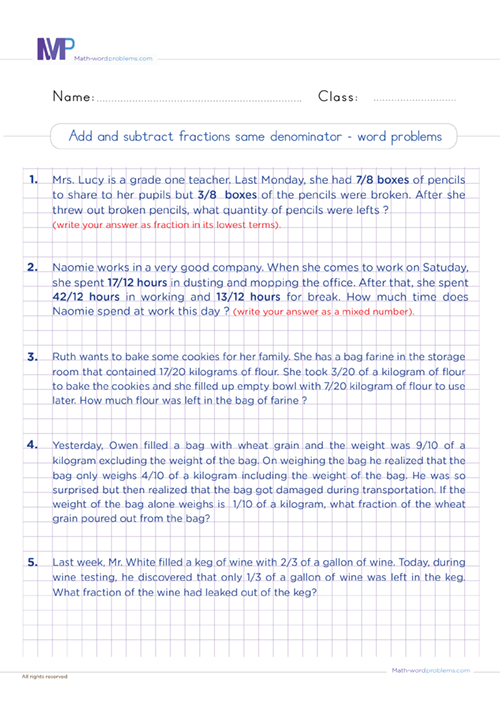
Adding and subtracting fractions and mixed numbers is as simple as adding and subtraction whole numbers. With fractions, you must ensure the denominators are the same before adding or subtracting. If the denominators are different, you'll need to find the least common denominator (LCD) and rewrite the fractions with equivalent denominators before adding or subtracting. As earlier said, you can convert mixed fractions to improper fractions before adding or subtracting.
Now, let's consider adding/subtracting mixed fractions with equal denominators, like 2 3/5 + 6 1/5
- Before we begin solving, you can see that we have a common denominator, 5
- Now, we will add the whole numbers separately, i.e., 2 + 6 = 8
- Secondly, we add the numerators, i.e., 3 + 1 = 4.
- So, our new numerator will be 4, while our denominator (5) remains the same.
- 2 3/5 + 6 1/5 = 8 4/5.
Subtracting mixed fractions follows the same procedure as above.
When multiplying mixed numbers, we must convert them to improper fractions first. If you are multiplying a mixed fraction by a whole number, rewrite the whole number as a fraction with the denominator 1. There, you'll have two fractions. This goes the same with multiplying fractions of a whole. Here, you simply need to change the "of" into a multiplication sign and multiply. For instance
When multiplying a fraction by a fraction (proper or improper), first multiply the two numerators and then multiply the two denominators. Finally, simplify the new fractions (answer) or convert it back to a mixed number if necessary.
When dividing mixed numbers, we must also convert the mixed numbers to improper fractions. If you are dividing a mixed fraction by a whole number, rewrite the whole number as a fraction with the denominator 1. There, you'll have two fractions. From here, you can follow the keep-change-flip method below:
- KEEP = Keep the first fraction as it is and leave it alone.
- CHANGE = Change the division sign to a multiplication sign.
- FLIP = Flip the second fraction (swap the numerator and the denominator)
Finally, solve the problem by multiplying the fractions and simplifying the answer or converting it back to a mixed number if necessary.
From the above methods of solving mixed fractions problems, you will bear with me that your kids will feel comfortable and confident in solving all fractions and mixed numbers problems, whether in math class or real life.
Strategies for quickly mastering fractions and mixed numbers worksheet with answers grade 5
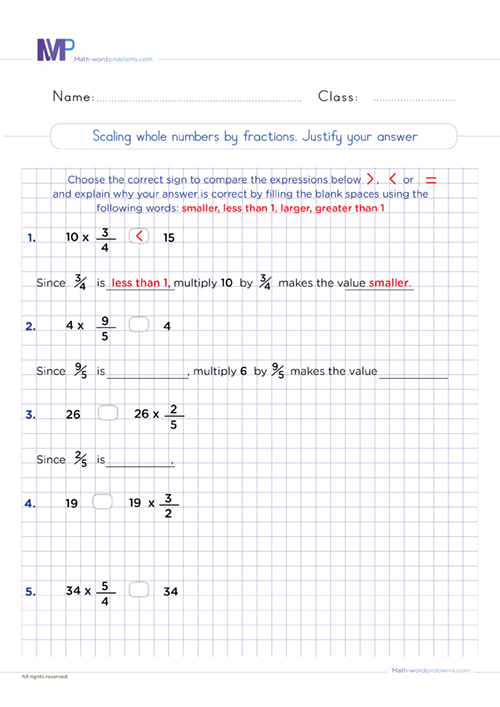
Are you in need of fun strategies for quickly mastering fractions and mixed numbers worksheet with answers grade 5? If yes! Then you are just at the right place. We will provide you with the best strategies to determine how big or small a fraction is. Unlike whole numbers, which, when we multiply, become bigger, fractions can become smaller, bigger, or even remain the same when we multiply them. As such, scaling is the best strategy to determine if a fraction is greater or less than a whole. In line with this, we will formulate easy-to-solve scaling whole numbers by fractions exercise by using the comparative signs (<, >, = ) to determine if an expression is smaller, bigger, or equal to a given whole.
Note the rules of scaling, which state that when you multiply by a fraction that is less than one whole, the size will be scaled down. Also, when you multiply by a fraction that is greater than one whole, the size will be scaled up. This rule will help kids determine the reasonableness of their answers when solving fraction problems.