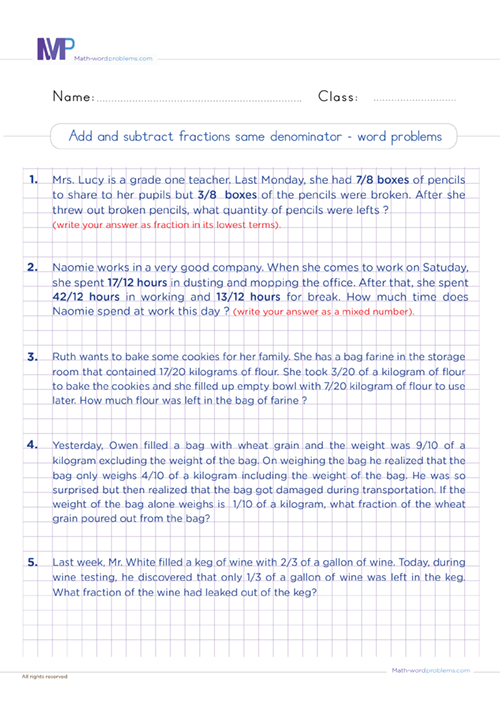
$>$ How to perform addition and subtraction of fractions with like denominators word problems

$Lesson summary$

Get more contents on this skill...

If you want to know how to perform addition and subtraction of fractions with like denominators word problems, then you are in the right place. Here is a captivating step-by-step guide on how to do it. These unique steps will build your kids’ maths word problem-solving skills and help them to provide accurate solutions whenever they encounter challenging problems.

Furthermore, this resource is perfect for learners because it doesn’t just concentrate on their ability to find the correct answer. It also strengthens their reading comprehension and analytical skills, i.e., logical thinking and ability to link real-world problems into imaginative word problems situations.

Steps on how to solve addition and subtraction of fractions with like denominators word problems

Our steps on how to solve addition and subtraction of fractions with like denominators word problems are short and straightforward. Most kids find it challenging when adding and subFor this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***tracting fractions with like denominators. We have a solution here for them.

We have designed unique and straightforward steps so kids can approach solving all word problems on addition and subtraction of fractions with like denominators in an enjoyable way.

In addition, we have designed exciting and familiar word problems with examples and a unique way of how incredible these steps work.

Step 1: IDENTIFY:

he most important thing here is to figure out the important fractions and keywords in the word problem. Then, use these keywords to identify whether the problem involves the addition or subtraction of fractions.

If the word problem requires you to add fractions, then you’ll likely encounter keywords such as:- add, plus, more, total, in total, increase, together, altogether, combined, sum, grow, join, both, in all, and, how many in all, how much, etc.
On the other hand, if the problem requires you to subtract fractions, you will see at least one of the keywords below in the word problem: - less, less than, excluding, minus, take away, left, decrease, difference, fewer, deduct, remain, change, how many more, left over, less than, how much longer/shorter, fewer than, discount, etc.

***One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***

Step 2: STRATEGIZE:

How will you solve or tackle the word problem?

Firstly, from the keyword(s) in the word problem, you will know if you need to add or subtract or perform any other operation.
Secondly, you must remember that, for addition and subtraction of fractions, all fractions must have the same denominators. But since you are already dealing with fractions with like denominators here, you are good to go.
Thirdly, remember you must not depend only on keywords. Instead, try to understand the situation that the problem is describing.
Finally, after knowing which operation you will perform, Construct short expressions/sentences to represent the given word problem.

Step 3: SET UP:

From the information you gathered from step 2, write down a numerical expression representing the information in the word problem.

Step 4: PROVIDE A SOLUTION:

From Step 3 above, add or subtract the fractions by adding or subtracting the numerators together while leaving the denominator the same. Furthermore, remember to include the unit of measurement if any. Also, simplify the fraction if possible.

Step 5: CHECK YOUR WORK:

In conclusion, ask yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.

$Find more resources here$

PRACTICE THIS SKILL NOW $Play$

Download worksheet

add and subtract fractions with like denominators

$add and subtract fractions with like denominators worksheet$

Print it

Examples on how to perform addition and subtraction of fractions with like denominators

Example one: Here is how to add fractions with like denominators.

In Carson’s sports club, of the members like volleyball, of the members like soccer and of the members like basketball. What fraction of the members like all the games?

Step 1:Sort out important fractions and identify the keywords found in the word problem.

The important fractions here are , , . The keyword identified here is “all.”

How do you solve the problem?

Step 2: From the keyword “all,” it is clear that the question is asking you to find the sum of the given fractions. Hence, this calls for an addition operation

Now, construct short expressions/sentences to represent

Fraction of the members that like basketball =
Fraction of the members that like soccer = = =
Fraction of the members that like volleyball =
herefore, the fraction of members that like all the games = the fraction of members that like basketball + the fraction of members that like soccer + the fraction of members that like volley ball.

Step 3: Now, write down a numerical expression to represent the bolded sentence in step 2 above:

+ +

Step 4: From step 3 above, since the denominators are the same add the numerators and leave the denominators the same. Also, simplify the fractions if possible. Always recall adding the unit of measurement to your final answer if any.

+ + = =

So, of the members like all the games.

Step 5: Lastly, check out your work by interpreting the answer in the context of the problem. If the interpretation makes sense then “YES”, you are done. If “NO”, go back to step 1 and start all over again.

Example two: how do you subtract fractions with like denominators word problems?

Rita has a ribbon that is of a meter long. She cuts out to decorate a gift for her friend. How much of the ribbon is left?

Step 1:First, you have to read the problem very well. After doing this, you’ll see that the important fractions here are, and . And the keyword found in the word problem is “left.”

Step 2: Next, how will you solve the problem? From the keyword “left,” it is clear that the question asks to find the difference of the given fractions. Hence, you have to perform a subtraction operation.

Now, after knowing which operation you will perform, construct short expressions/sentences to represent the given word problem.

Fraction of a meter of ribbon that Rita has =
Fraction of a meter of ribbon that she cut =
Therefore, the fraction of the ribbon left = the fraction of a meter of ribbon that Rita has – the fraction of a meter of ribbon that she cut.

Step 3: Then, write down a numerical expression to represent the bolded sentence in step 2 above:

- = ?

Step 4: From step 3 above, since the denominators are the same, simply subtract the numerators and leave the denominators the same. Also, simplify the fractions if possible. Furthermore, add the unit of measurement to your final answer if any.

- = =

So, of a meter of ribbon is left

Step 5: Finally, check out your work by interpreting the answer in the context of the problem. If the interpretation makes sense then “YES”, you are done. If “NO”, go back to step 1 and start all over again.

$>$ How to perform addition and subtraction of fractions with like denominators word problems

Get more contents on this skill...