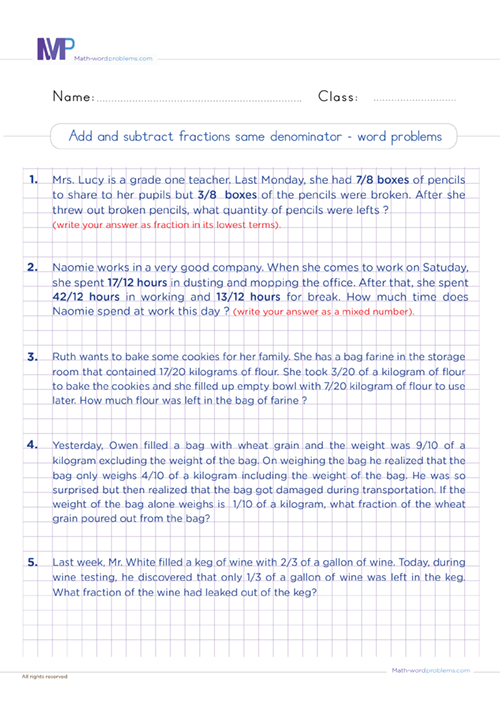
Strategies to use when adding and subtracting fractions with unlike denominators word problems
Strategies to use when adding and subtracting fractions with unlike denominators word problems

- INTRODUCTION
- Step 1 IDENTIFY
- Step 2 STRATEGIZE
- Step 3 SET UP
- Step 4 PROVIDE A SOLUTION
- Step 5 CHECK YOUR WORK
Get more contents on this skill...
Have you been looking for simple strategies to use when adding and subtracting fractions with unlike denominators word problems? Look no further. This fantastic resource will direct your kids on how to do it.
Our reason for designing captivating strategies for 6th graders is to reinforce their logical thinking and fraction skills. Suppose your kids can adequately follow these guides and strategies of tackling fractions with unlike denominators word problems on addition and subtraction keenly, then be confident that they will be able to solve all fractions and other mixed math problems accurately and confidently.
Steps on how to Solve Addition and Subtraction of Fractions with Unlike Denominators Word Problems
The stunning steps on how to solve addition and subtraction of fractions with unlike denominators word problems below will enable kids to tackle any problem they come across, no matter how challenging it seems.
However, we have attached enriching and fun examples at the end of this resource for practice purposes and to show kids how magical these steps work.
Step 1: IDENTIFY:
Then, use these keywords to identify whether the problem involves addition or subtraction.
- Suppose the word problem requires that you add fractions with unlike denominators, you will see at least one of the following keywords: - add, plus, more, total, in total, increase, together, Altogether, combined, sum, grow, join, both, in all, and, how many in all, how much, etc.
- But suppose you need to subtract fractions with unlike denominators, you will see at least one of the keywords below in the word problem: - less, less than, excluding, minus, take away, left, decrease, difference, fewer, deduct, remain, change, how many more, left over, less than, how much longer/shorter, how much farther, fewer than, discount, etc.
- ***One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
- For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***
Step 2: STRATEGIZE:
Now, how will you solve or tackle the word problem?
- As mentioned earlier, from the keyword(s) in the word problem, you will find out if you need to add or subtract or perform any other operation.
- Also, for addition and subtraction of fractions, all fractions must have the same denominators. If your fractions already have the same denominator, then you are good to go. If not, you must find the lowest common denominator (LCD). Then write equivalent fractions with the LCD you just found by multiplying the given fractions by a suitable number in order to achieve fractions with the same denominator.
- Most importantly, remember you must not depend only on keywords. Rather, try to understand the situation that the problem is describing.
- Last but not the least, after knowing which operation you are going to perform, construct short expressions/sentences to represent the given word problem.
Step 3: SET UP:
This step will require you to write down a numerical expression representing the information in the word problem.
Step 4: PROVIDE A SOLUTION:
Here, since you are dealing with unlike denominators, you need to find the LCD and write equivalent fractions with the LCD you just found. i.e., multiplying the given fractions by a suitable number to achieve fractions with the same denominator.
Then, add or subtract the fractions by adding or subtracting the numerators together while leaving the denominator the same.
Also, simplify the fraction if possible. Note that you should always include the unit of measurement in your final answer, if any.
Step 5: CHECK YOUR WORK:
To conclude, ask yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.
Examples on the strategies to use when adding and subtracting fractions with unlike denominators word problems
Example one: How to subtract fractions with unlike denominators word problems.
Step 1: First, after reading the problem, the important fractions that you will come across are
Step 2: As we move to this step, this is how you will solve the problem. You see that, from the situation that the problem is describing and the keyword found in the word problem, it is evident that the question asks to find the difference of the given fractions. Hence, this calls for a subtraction operation.
Now, after knowing the operation you will perform, Construct short expressions/sentences to represent the given word problem.
- Distance he usually covers every day =
of a mile - Distance he has already covered =
of a mile - Therefore, the distance left to be covered = the distance he usually covers every day – the distance he has already covered.
Step 3: Now, write down a numerical expression to represent the bolded sentence in step 2 above:
Step 4:From step 3 above, since the denominators are not the same, find the LCD of 4 and 2.
Then, write equivalent fractions with the LCD you just found. i.e., multiplying the given fractions by a suitable number to achieve fractions with the same denominator.
After that, add the fractions by adding the numerators together while leaving the denominator the same.
Also, simplify the fraction if possible.
Lastly, always add the unit of measurement to your final answer if any.
Since 4 and 2 are both factors of 4, you can use 4 as the least common denominator)
So, he has to walk
Step 5:Finally, check out your work by interpreting the answer in the context of the problem. If the interpretation makes sense, then “YES,” you are done. If “NO,” go back to step 1 and start all over again.
Example two: How do you solve addition and subtraction with unlike denominators word problems?
Step 1: First, after reading the problem, the important fractions that you will find are
Step 2:Next, how will you solve the problem? From the keyword “altogether” and the situation that the problem is trying to describe, it is clear that the question asks to find the sum of the given fractions. Hence, this is an addition operation.
Then, after knowing which operation you will perform, Construct short sentences to represent the given word problem.
- Quantity of a cake Perez ate =
- Quantity of a cake Jones ate =
- Quantity of a cake Jack ate =
- Therefore, the quantity of cake they ate altogether = the quantity of cake Perez ate + the quantity of cake Jones ate + the quantity of cake Jack ate.
Step 3: Now, write down a numerical expression to represent the bolded sentence in step 2 above:
Step 4: From step 3 above, you’ll discover that the denominators are different. Hence, find the LCD of 5, 2, and 7.
Then, write equivalent fractions with the LCD you just found. I.e., multiply the given fractions by a suitable number to achieve fractions with the same denominator.
After that, add the fractions by adding the numerators together while leaving the denominator the same.
Also, simplify the fraction if possible.
Lastly, always add the unit of measurement to your final answer, if any.
(Since 5, 3, and 7 are all factors of 105; you can use 105 as the least common denominator)
Step 5: