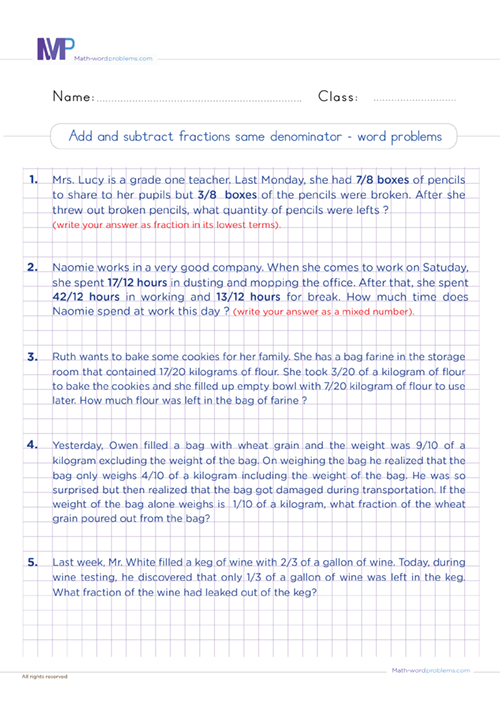
$>$ What is the easiest way to add and subtract mixed numbers in word problems?

$Lesson summary$

Get more contents on this skill...

Have you ever asked yourself what is the easiest way to add and subtract mixed numbers word problems? Well, here is a powerful, simple step-by-step guide to getting kids excited about solving these challenging yet enriching real-life grade six word problems.

Amazingly this guide has the best techniques that kids will use to have a quick mastery of word problems, no matter how complex they may seem. Furthermore, the fundamental purpose of this creative guide is to make the critical thinking required for solving word problems easier.

Steps to Solve Addition and Subtraction of Mixed Numbers Word Problems

We’ve got here remarkable steps to solve addition and subtraction of mixed numbers word problems quickly. This will make learners not only attempt every word problem they encounter but will provide complete and accurate solutions to word problems they will encounter with ease.

Furthermore, our main aim is to provide kids with the most straightforward yet technical strategy they can use to become great, skillful geniuses in solving math word problems.

Also, we’ve added real-life examples/situations to show kids how easy and fulfilling these steps are.

Step 1: IDENTIFY:

To identify or figure out the important mixed numbers and keywords in the word problem, you have to read the problem very well and understand it. Then use these keywords to identify whether the problem involves addition or subtraction.

When it comes to adding mixed numbers word problems, the keywords you’ll likely come across are: - add, plus, more, total, in total, increase, together, Altogether, combined, sum, grow, join, both, in all, and, how many in all, how much, etc.
Similarly, concerning subtracting mixed numbers word problems, these are the keywords you should expect to see in the word problem: - less, less than, excluding, minus, take away, left, decrease, difference, fewer, deduct, remain, change, how many more, left over, less than, how much longer/shorter, how much farther, fewer than, discount, etc.

***One key Element for learners to understand is that they should not always rely on keywords alone. That is to say; the same keyword can have different meanings in different word problems.
For this reason, we reiterate on the importance of reading the question very carefully to understand the situation that the word problem is describing, then figure out exactly which operation to use***

Step 2: STRATEGIZE:

How will you decode or tackle the word problem?

You will know from the keyword(s) in the word problem if you need to add or subtract or perform any other operation.
Also, for addition and subtraction of mixed numbers, all fractions must have the same denominators. If your fractions already have the same denominator, then you are good to go. If not, you have to find the lowest common denominator (LCD) and write equivalent fractions with the LCD you just found. i.e., multiplying the given fractions by a suitable number to achieve fractions with the same denominator.
Remember that you must not always depend only on keywords. Rather, try to read and understand the situation that the problem is describing.
So after knowing the exact operation you will perform, Construct short expressions/sentences to represent the given word problem.

Step 3: SET UP:

Here, write down a numerical expression/ mathematical equation representing the information given in the word problem.

Step 4: PROVIDE A SOLUTION:

From Step 3 above, do the following to solve the word problem.

Firstly, add or subtract the whole numbers in both mixed numbers together.
Secondly, if the denominators of the mixed numbers are the same, simply add the numerators while keeping the denominator the same.
Thirdly, if the denominators are not the same, find the LCD and write equivalent fractions with the LCD you just found by multiplying the given fractions by a suitable number in order to achieve fractions with the same denominator. Then, go ahead and add or subtract the fractions by adding or subtracting the numerators together while leaving the denominator the same.
Also, simplify the fraction if possible.
Finally, do not forget to add the unit of measurement to you final answer if any.

Step 5: CHECK YOUR WORK:

To finalise, ask yourself this question. “Does my answer make sense?” If “YES,” you are done. If “NO,” go back to step 1 and start all over again.

$Find more resources here$

PRACTICE THIS SKILL NOW $Play$

Download worksheet

Add and subtract mixed numbers word problems

$Add and subtract mixed numbers word problems worksheet$

Print it

Examples on how to solve addition and subtraction of mixed numbers word problems.

Example one: How to subtract mixed numbers word problems.

Allan owns 50 hectare of farm in his community. His friend is into farming too and owns 87 hectare of farm in his own community. How much more hectare of farm does his friend has than him?

Step 1: After reading the problem very carefully, these are the important mixed numbers that you’ll find 50 and 87. Also, the keyword found in the word problem is “how much more”.

Step 2: Next, how will you solve the problem? From the scenario that the problem is presenting and the keyword “how much more”, it is clear that the question asks to find the difference of the given mixed numbers. Hence, this is a subtraction operation.

Then, after knowing which operation you are going to perform, create short expressions/sentences to represent the given word problem.

Hectares of farm Allan has = 50
Hectares of farm his friend has = 87
Therefore, the hectares of farm his friend has more than him = the hectares of the farm his friend has – the hectares of farm his friend has.

Step 3: Now, write down a numerical expression/a mathematical equation to represent the bolded sentence in step 2 above:

87 - 50 = ?

Step 4: From Step 3 above, do the following to solve the mathematical equation representing the word problem.

Firstly, subtract the whole numbers in both mixed numbers together.
Secondly, since the denominators of the mixed numbers are the same, just go ahead and subtract the numerators while keeping the denominator the same.
hirdly, simplify the fraction if possible.
Lastly, remember to include the unit of measurement to your final answer if any

87 - 50 = (87-50) = 37

So, his friend has 37 more hectares of farm than him

Step 5: Finally, check out your work by interpreting the answer in the context of the problem. If the interpretation makes sense, then “YES,” you are done. If “NO,” go back to step 1 and start all over again.

Example two: How to subtract mixed numbers word problems.

Dickson has 4 boxes of candy. If he eats 1 of it, how many boxes of candy does he have left?

Step 1: First, the important mixed numbers found after reading the problem and understanding it very well are 4 and 1. Now, the keyword found in the word problem is “left.”

Step 2: Next, how will you solve the problem? From the keyword “left,” it is clear that the question asks to find the difference between the mixed numbers. Hence, you have to perform a subtraction operation.

Then, after knowing which operation you will perform, Construct short expressions/sentences to represent the given word problem.

Boxes of candy he bought = 4
Boxes of candy he ate = 1
Therefore, the number of boxes of candy he has left = the boxes of candy he bought – the boxes of candy he ate.

Step 3: Now, write down a numerical expression to represent the bolded sentence in step 2 above:

4 - 1 = ?

Step 4: From Step 3 above, do the following to solve the word problem.

Firstly, subtract the whole numbers in both mixed numbers together.
Secondly, since the denominators are different, find the LCD of 4 and 5. Write equivalent fractions with the LCD you just found. i.e., by multiplying the given fractions by a suitable number to achieve fractions with the same denominator.
Thirdly, subtract the fractions by subtracting the numerators together while leaving the denominator the same.
Fourthly, simplify the fraction if possible.
Lastly, remember to include the unit of measurement in your final answer if any

(Since 5 and 4 are all factors of 20; you can use 20 as the least common denominator)

4 - 1 = (4-1) = 3 = 3

So, he has 3 boxes of candy left

Example three: How to solve addition of mixed numbers word problems.

Mason is practicing water therapy. In the morning, he drank 2 liters of water. In the afternoon, he drank 4 liters of water. In the evening, he drank just 1 liter of water. How many liters of water did he drink in all?

Step 1: First, after reading the question well, the important mixed numbers here are 2, 4 and 1 and the keyword found in the word problem is “in all.”

Step 2: Next, how will you solve the problem? From the keyword “in all” found in the word problem and the situation that the problem is describing, it is clear that the question asks to find the sum of the given mixed numbers. Hence, you have to carry out an addition operation.

After knowing which operation you will perform, construct short expressions/sentences to represent the given word problem.

Quantity of water he drank in the morning = 2 liters = 2 = 2
Quantity of water he drank in the afternoon = 4 liters
Quantity of water he drank in the evening = 1 liter
Therefore, the quantity of water they drank in all = the quantity of water he drank in the morning + the quantity of water he drank in the afternoon + the quantity of water he drank in the evening.

Step 3: Now, write down a numerical expression/ a mathematical equation to represent the bolded sentence in step 2 above:

2 + 4 + 1 = ?

Step 4: Then, from Step 3 above, do the following to solve the word problem.

Firstly, add the whole numbers in both mixed numbers together.
Secondly, since the denominators of the mixed numbers are the same, add the numerators while keeping the denominator the same.
Thirdly, simplify the fraction if possible.
Lastly, remember to include the unit of measurement to your final answer if any

2 + 4 + 1 = (2+4+1) = 7 = 7 = 7 + (1) = 8

So, he drank 8 liters of water

Example four: How to solve addition of mixed numbers word problems.

Frank used 5 cups of water and 1 cup of oil to cook rice. How many cups of water and oil did he use in all?

Step 1: Start by reading to understand the problem and question very well. Then, pick out the important mixed numbers, which are 5 and 1 . As you read further, you’ll find out that the keyword in the word problem is “in all.”

Step 2: Then, how will you solve the problem? From the keyword “in all” found in the word problem and the scenario that the problem has presented, it is clear that the question asks to find the sum of the given mixed numbers. Hence, this calls for you to perform an addition problem.

Now, after knowing which operation you will perform, Construct short expressions/sentences to represent the given word problem.

Number of cups of water he used = 5
Number of cups of oil he used = 1
Therefore, the number of cups of water and oil he used in all = the number of cups of water he used + the number of cups of oil he used.

Step 3: Next, write down a mathematical equation to represent the bolded sentence in step 2 above:

5 + 1 = ?

Step 4: From Step 3 above, do the following to solve the word problem.

Firstly, add the whole numbers in both mixed numbers together.
Secondly, since the denominators are different, find the LCD of 6 and 4. Write equivalent fractions with the LCD you just found by multiplying the given fractions by a suitable number to achieve fractions with the same denominator.
Thirdly, add the fractions by adding the numerators together while leaving the denominator the same.
Fourthly, simplify the fraction if possible.
Lastly, remember to include the unit of measurement in your final answer if any

(Since 6 and 4 are all factors of 24; you can use 24 as the least common denominator)

Next, write down a mathematical equation to represent the bolded sentence in step 2 above:

5 + 1 = (5+1) = 6 = 6 = 6 = 6

So, he used 6 cups of water and oil to cook rice in all.

$>$ What is the easiest way to add and subtract mixed numbers in word problems?

Get more contents on this skill...